18
Carla waited quietly at the entrance to Assunto’s office until he looked up from his work and gestured for her to enter. “There’s good news and there’s bad news,” she announced as she dragged herself toward his desk. “But best of all, there’s a chance to make the bad news good.”
Assunto managed a weary buzz. “Why can’t things ever be simple with you?”
“I make them as simple as possible,” Carla replied. “But no simpler.”
“So tell me the good news.”
Carla took a sheet of paper from her pocket and handed it to him.

“This is what happens when you take a luxagen with access to just two energy levels and hit it with a beam of light at a frequency tuned to the difference between those levels.”
Assunto stopped her. “What does that mean? ‘Tuned to the difference’?”
“Ah.” Carla realized that it had become second nature to her to think of energies and frequencies as interchangeable. She had to make a conscious effort now to unpack the details behind the instinctive translation. “If you imagine a particle and a wave moving at the same speed, the energy of the particle will be proportional to the frequency of the wave—with the ratio unchanged as you vary their common speed. If you set the speed to zero, the ratio is the mass of the particle divided by the maximum frequency of the wave—and that’s what it remains for every other speed.”
“That’s just geometry!” Assunto said. “The wave’s propagation vector will be parallel to the particle’s energy-momentum vector. That locks all of their components into a fixed ratio with each other.”
Carla said, “Yes—but now go a step further and suppose that the same ratio holds for every wave and its corresponding particle, whether it’s a luxagen wave and a luxagen or a light wave and a photon. None of the physics makes sense unless this ratio is a universal constant; I think of it as ‘Patrizia’s constant’, because the whole idea started with her. It’s as if these particle masses really are the maximum frequencies of the corresponding waves… just measured in different units.”
Assunto looked pained for a moment, but then he said, “You mean like times and distances?”
“Perhaps.” Carla didn’t want to over-reach with the comparison: one was a fundamental truth about the cosmos that the Peerless itself had helped to prove beyond doubt; the other was an appealing, but still untested, speculation.
Assunto said, “So let’s take it for granted that we can turn any frequency into an energy, and vice versa. You have a luxagen trapped in some energy valley, and the corresponding wave equation has two solutions with definite frequencies.”
“Yes,” Carla replied. “What I’ve drawn for the two waves is their variation in space, but while maintaining that shape they’re oscillating in time, each one with its own pure frequency.”
“Then you add a light wave whose frequency matches the difference between the luxagen frequencies—and it drives the low-frequency luxagen wave up to the higher frequency?”
“Yes.”
“Well, that much makes sense,” Assunto said. “You can do something similar with waves on a string, if you vary the tension periodically at a frequency equal to the difference between the frequencies of two resonant modes.”
“What’s more surprising, though,” Carla said, “is the simple rule that this wave follows along the way. Where I’ve plotted the proportion of each of the waves, the arc that links the point that’s ‘purely wave one’ to the point that’s ‘purely wave two’ isn’t an artistic flourish: the dynamics really does follow a perfect circular arc. The sum of the squares of the two proportions remains equal to one, throughout the process.”
“I see.” Assunto was prepared to take her word for this, even if the significance of it escaped him.
Carla said, “Hold on to that thought.”
She produced the second sheet.

“I take it that this is the bad news.” Assunto examined the diagram. “The light never frees the luxagen? So… that’s the end of your theory of tarnishing?”
“Wait!” Carla pleaded. “When there are just two waves, two energy levels, you’d expect the dynamics to take you all the way from one pure wave to the other. Where else are you going to go? But here, there are a multitude of free waves whose frequencies are almost identical—what I’m showing on the vertical axis covers them all. So there are ways you can wander around in this space of possibilities—keeping the sum of the squares of the proportions equal to one, as before—without the trapped-wave proportion ever falling to zero.”
“Without it ever falling very far at all,” Assunto noted, pointing to the modest arc that showed the limits of the process. “Which I can well believe, given your assumptions. But why isn’t it fatal? How can this be a description of light knocking a luxagen out of its valley, if the wave barely changes no matter how long you expose it to the light?”
Carla braced herself. She had managed to convince Patrizia and Onesto that her hypothesis wasn’t entirely deranged, but Assunto would be the real test.
“The thing is,” she said, “there’s always more than one luxagen and a light wave to consider. There’s the whole slab of mirrorstone as well. We can sum up most of its influence in terms of a simple ‘energy valley’, but the reality is more complicated than that. With all the luxagen waves reaching part-way out of their own valleys, every luxagen is interacting with its neighbors—and to some degree with its neighbors’ neighbors, and so on.”
“So your model’s inadequate?” Assunto suggested.
“Yes,” Carla conceded. “But a model of the entire solid would just be intractable. The only way we can get anywhere is to try to find a rule of thumb that lets us extract useful predictions from the things we can model.”
Assunto was skeptical. “What kind of rule?”
“We start with two reasonable assumptions,” Carla said. “If a wave that is purely trapped interacts with the rest of the solid, it remains trapped. If a wave that is purely free interacts with the rest of the solid, it stays free.”
Assunto said, “I can live with that. But what happens to a mixture of the two?”
“I doubt we could ever predict that with certainty,” Carla admitted. “Not without knowing exactly what’s going on with every single luxagen in the solid. But maybe we can still predict what will happen on average. If we treat the square of the proportion of the wave that’s trapped as the probability that the luxagen will remain trapped when it interacts with the rest of the solid, everything makes sense—because the squared proportions always add up to one, just as the probabilities for any set of alternatives always add up to one. I know it sounds too simple to be true—but the mathematics seems to be offering us the perfect number to use as a probability when we can’t make an exact prediction.”
Assunto raised a hand for silence, and Carla let him think the whole thing over. Finally he said, “When, exactly, does this probability get turned into a fact? You have the luxagen wave changing shape under the influence of the light alone, but then at some point it’s supposed to interact with the rest of the solid, which finally determines its fate. But the probability keeps changing, as the wave changes shape. So what probability do you use?”
Carla said, “It makes no difference exactly when the interaction happens, so long as it happens often enough, and so long as the probability grows in direct proportion to the time. Suppose the probability is one in a gross after one pause, two in a gross after two pauses, and so on. If the rest of the solid only interacts with each luxagen once every pause, the rate of tarnishing will be one luxagen per gross per pause. But even if the interaction takes place far more frequently than that, each time it happens the probability will have risen to a much smaller value than it would have reached if the luxagen had been left undisturbed for longer. The two effects—the lower probability and the greater number of interactions—almost cancel each other out, and you end up with a simple exponential decay curve.”
She sketched the result.
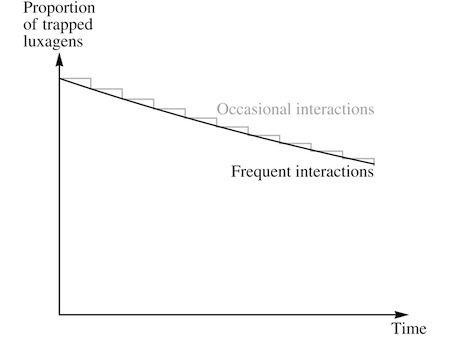
Assunto was not impressed. “Almost every process looks linear on a short enough time scale, so whatever’s going on with the tarnishing the net result could end up looking like exponential decay. If I gave you the sunstone for one more experiment, and you came back to me with a curve like that, what would it prove? Nothing.”
“One curve would be meaningless,” Carla agreed. “But this is where the bad news finally redeems itself. When the energy gap is small enough for a light wave to bridge the two frequencies, the rate at which the probability grows is just proportional to the intensity of the light. But the tiers we found with the mirrorstone suggest that the energy gap is four times too big for that—and for lower frequencies of light, five times too big. In which case, the rate is no longer proportional to the intensity itself: it’s proportional to the fourth or fifth power.”
Assunto grasped the significance of this immediately. “So it’s a higher-order effect,” he said. “The light wave creates a small disturbance in the energy valley, and the effect of that isn’t perfectly linear—so a complete description would have to include ever-smaller terms that depend on the square of the wave, the cube, the fourth power…”
“And the fourth power of the wave,” Carla added, “contains a frequency four times higher than that of the wave itself. There is no light with a frequency high enough to bridge the energy gap in a stable solid—but the fourth power of the same disturbance oscillates four times faster.”
“So how are you proposing to test all this?” Assunto pressed her.
“In the past, I’ve wasted sunstone,” Carla admitted. “There were things I could have measured that I didn’t even try to record. This time I’ll do it properly, once and for all. With a system of apertures and shutters, in a single run I can expose different parts of the same slab of mirrorstone to different intensities of light, for different lengths of time. The variation in the tarnishing over time should give us the exponential decay curves—and the variation with intensity should confirm the fourth-power rule in the first tier, and the fifth-power rule in the next. If we do find those power rules, surely that will be a sign that we’re on the right track.”
Assunto said, “Last time, the tiers were meant to mark the number of ‘photons’ each luxagen needed to make in order to break free.”
“They still do!” Carla replied. “These powers of the light’s intensity are the only way I know to calculate the tarnishing rates, but that doesn’t mean photons are out of the picture. When a luxagen changes its energy level, it still has to add a whole number of photons to the light: four or five, just as before.”
“But what drives the luxagen from one level to another in the first place?” Assunto answered the question himself. “Not a bombardment with particles, but the shaking of a wave.”
Carla couldn’t deny that. Patrizia’s interpretation of the scattering experiment in terms of colliding particles seemed irrefutable, but as yet there was no way to describe the tarnishing in the same language. They were still groping their way toward the truth, and the argument everyone had once thought settled in the days of Giorgio and Yalda was refusing to lie quietly in its grave.
Assunto said, “I’ll give you the sunstone for one more experiment, but that’s it. No more tinkering with the theory and trying again. If you don’t find the power rules you’ve predicted, you’ll have to accept that your ideas have been refuted and move on. Agreed?”
Carla had known that they were approaching this point, but to hear it put so starkly gave her pause. She could return to her collaborators and work through everything one more time: checking their calculations, revisiting their assumptions. Maybe they’d missed something crucial that would lead them to change their predictions—or something that could sweep away the lingering confusion and provide them with a surer bet.
But in less than two stints, Assunto would be answering to an entirely new Council, and there was no guarantee that he’d still have the power to offer her any sunstone at all. If they ended up losing the chance to perform this last experiment, there were no calculations that could tell them whether or not they’d been wasting their time. They needed to know the result itself, even more than they needed to be right.
Carla said, “Agreed.”
 ТЕЛЕГРАМ
ТЕЛЕГРАМ Книжный Вестник
Книжный Вестник Книжный Бот
Книжный Бот Любовные романы
Любовные романы Саморазвитие
Саморазвитие Детективы
Детективы Фантастика
Фантастика ВКОНТАКТЕ
ВКОНТАКТЕ